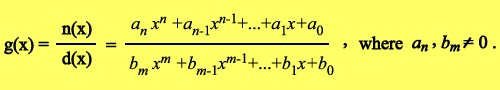
Horizontal asymptotes occur when the values of a given function, say g(x),
approach a finite number as x
® +¥ or
-¥. In our example, the function was f(x) and that finite number was 0.
Determining horizontal asymptotes
for a given rational function is very easy. Let's have a function
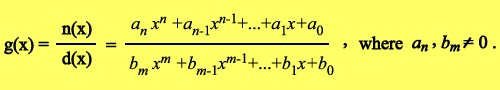
Since we are interested in the behavior of g(x) for x very large, or for x very negative (that is, negative of large magnitude), terms containing x in lower powers matter very little as they increase in magnitude much slower than the highest terms. Hence, for x's close to plus or minus infinity, g(x) behaves more or less like the quotient of the highest terms of the numerator and the denominator:
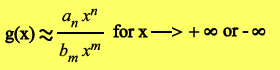
The latter quotient determines horizontal asymptotes of g(x).
 |
 |